How to Get a Moron out of a Tree

[6/1: edited slightly. still opaque.]

Vaguely, this post is based on a stimulating comment at Unqualified Offerings that I read several weeks or months ago (on a post about physics education, which limits it to maybe 1 in 3, and by someone who's obviously a more enthusiastic sort of nerd than I am, which also doesn't narrow things down very much--naturally, I couldn't track down the original, so when the time comes, just assume I'm paraphrasing someone who knew what they were talking about), and the fact that this particular month, I'm busy feigning expertise in the gray areas between physical optics and acoustics. Now, if I were a better scientist, I might get worked up about some clever solution, or about some amazing counterintuitive result, or an impressive discovery, or I might keep myself up nights dreaming about my own rudimentary maths or Rube Goldberg apparati, and okay, sometimes I do those things, but what really tends to impress me are the things that good scientists have long since chalked up and left behind for the challenging problems--it's the remarkable fact that any of this shit works at all.

(And perhaps it's an appropriate moment to explore here the raison d'etre of this-here blog, if, in fact, there is one. I update much too rarely, and I'm never sufficiently on any particular point to grab readers (limited politics), and this particular sort of post has just got nothing for anyone. It's below the level of people who would know what they're doing in these fields, and it is yet too pointlessly geeky to entice any hypothetical passersby. It's as if I'm getting giddy over the overview chapter in my (company's) Optics book. But I admit that this is a triviality that I find satisfying and slightly amusing. And since I was motivated enough to draw a picture today, there could be no going back. Somebody please wave to that poor guy.)

The commenter in question stated that one potentially great thing to teach budding scientists would be the idea that not every goddamn term in the expansion is physically significant. "Yes," I thought at the time. "Damn straight." I had a vivid recollection of some group meeting back when where some solution of somethingorother resulted in several terms, and the authors went out of their way to make up meaning for each of them, independent of any theoretical justification. I had a hard time that day expressing just what it was that irritated me about the work, and here it was, years later, with the sort of pith I appreciate.

Mathematical series expansions are important tools, don't get me wrong. They come up in the physical sciences in an effort to simplify nonlinear problems, or else as solutions to the equations governing wave motion. Through them, you can generate functions represented as sums of other (ideally easier) functions, but is that a real physical picture? Are waves made up of actual little wavelets? Are those squared and cubed terms really physically significant in their own right?

I recall power series in various sorts of chemical engineering applications. For example, a useful equation of state (relating temperature, pressure and density of some fluid) generally varies from the simple ideal gas law, and one way to account for this is a power series expansion on one of the parameters, to get successively more accurate approximations with every term. [A general power series looks like A₁x + A₂x² + A₃x³ + ... on out forever. Generally there's a mathematical formula to derive the coefficients if you're trying to increase the accuracy of your function of x, but for physical reality, you can go with just fitting curves too, and in ideal cases terms will fall out of physical theory, possibly allowing for some fudge factors.] I don't know if it started with Van der Waals, but his corrections to the ideal gas law used the most intuitive ideas (molecules take up space), and it generated a few extra terms, much like a formal expansion would. Virial equations of state (as they came to be called) were developed based first on just the convenient mathematical expansion, but eventually the terms were assigned physical significance based on statistical mechanics. I like that they were made to mean something, but I don't really believe that any significant data could be made on for those high-order interactions with the tiny coefficients.

In science, Fourier series, which are sums of sines and cosines (and which add harmonics instead of powers, A₁sin x + A₂sin 2x + ...), get a lot more traction than power series do. There are a lot of reasons for this, most fundamentally because a lot of natural phenomena oscillate. Waves are everywhere (everywhere! thanks, Schmutzie), from sound to vibrating solids to light, and trigometric functions, which like to bob up and down, are an obvious way to represent them. Not that natural waves are always exactly sinusoidal, but most any periodic function can be represented by a Fourier series with enough terms. When you add periodic functions, you get new periodic functions, which is a handy thing when you want to describe how acoustic or electromagnetic waves interact with one another. The smaller waves superpose (i.e., add) to create some other wave, also represented by some Fourier series, whose properties it may be useful to consider separately. Conversely, some wave pattern can be imagined to be the sum of a whole lot of smaller wave patterns.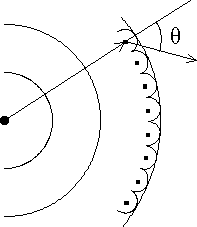

One of the basic ideas in optics--and one of the student's first what-the-fuck moments--is Huygens' principle, which he imagined as a physical explanation. Huygens averred that a propagating wave--whether in matter or (as he thought of it) the luminiferous aether--was in fact the sum of a whole bunch tiny spherical wavelets, which could be thought to exist at every point in the advancing phase front, which all superposed to form the wave as it was observed. As the wave progressed, new wavelets would be generated and propagated forward too. It went far (and goes far) to describe phenomena like diffraction of light and other waves when it passes through an aperture.

This was in the seventeenth century, by the way, contending some of Newton's famous points, and it's interesting that the physical interpretation apparently came first. (Maybe I should check this? People were monkeying with infinite series by then and maybe it informed the idea?) Auguste Fresnel (remember him?) fixed one of the big basic hangups before too long (spherical wavelets needed some justification for not radiating backwards as well as forwards), but it took quite awhile before Huygens' principle was shown to satisfy a general wave equation for propagation (with, I believe I've read, some dubious boundary conditions). And yeah, that's the other great thing about Fourier series: harmonic functions turn out to be solutions for any number of differential equations that you can derive for mechanical motion or electromagnetism. These predict the oscillatory phenomena that appear throughout classical physics, and as you might imagine, a solution that can be represented by a Fourier series, can be represented by more than one Fourier series, or by sums of Fourier series. But that's math. As a physical matter, this Huygens thing remains pretty brazen: sure you can add up the cosines, but is a real wave one oscillation and a zillion wavelets both at the same time?

Huygens' principle has had its doubters, a quiet dissent that's evidently (with a little background reading) persisted. Einstein dismissed any significance of wavelets beyond a mathematical convenience. And Feynman thought that it wasn't the right physical picture either, although by then he was advancing the quantum mechanical nature of light: not wavelets, he argued, but probability distributions, which then sum up to physical observation. (Quantum mechanics can probably be taken as a more "real" picture for the action of fundamental particles, but this idea of wavelets helps out in diffraction of mechanical waves too, which don't need quantum. Quantum mechanics, at least as it applies to electrons in matter, likes to take on (sums of) harmonic functions too, and the huge conceptual leap there is that it evolves probability distributions instead of any clear picture of motion. Which isn't so bizarre until you try to wrap your head around the idea that these fundamental particles are odd physical things. I won't pretend to any useful understanding of QED, however.)

When waves travel through some medium, they cause sympathetic disturbances within it, and any amount of localized funny business ensues, propagating waves interacting with the vibrations they excite in the first place. (It occurred to me recently that I've spent years now, off and on trying to artificially create physical media that capture the quantum or the classical dynamics explicitly, on larger-than-usual scales.) Classical wave theory can get you pretty far even in these modern times. Generally speaking, it gets more challenging the more the waves interact with their media, and there are any number of degrees of description between, say, Maxwell's equations in free space and an entirely quantum picture. Acoustics generally works fine only considering matter as a continuum (but quantum vibrations exist, which matter in some fields).

When waves in media get intense, they can alter the properties matter as they pass through it, which can make the (classical) physics a lot harder. I don't know much about nonlinear optics, but when I've dabbled with nonlinear acoustics, second-order terms in various power series could be developed to explain those high-amplitude phenomena--you take another expansion to bring those second-order effects into reality. (Coincidentally enough, adding up powers of cosines, which you might do for that nonlinear acoustics problem, is basically the same thing as adding up harmonics of cosines as in a Fourier series, by virtue of some basic trigonometric identities. "Second order" often means the same thing in a conversational sense.) So a second harmonic can be teased out of the power series, a double-fast wave which the superposition principle tells us could have really been there all along. Sure, it's the next-best mathematical formula, but damned if the frequency double that evolves in a real measurement doesn't look a pure thing all by itself. Was it there lurking under there under the primary wave all along?

(How to get a moron out of a tree? Wave to him. Get it?)

Roamer, Wanderer, Nomad, Vagabond

My father sometimes requests that, should the unlikely combination of loneliness and health occur simultaneously late in his life, he simply be released into the wild. From some dark hidey-hole in the shrinking Connecticut backwoods, he'll range around, tramp on needles and leaves, and wear a circuit back and forth to his favorite places, emerging occasionally from the boughs to accept charity, scare children, or tell stories to willing listeners. His clothing will tatter--he'll definitely have to get a hat for the ensemble--and his beard will get appropriately bushy and twig-filled as he takes on the role of Local Color, a rarely-glimpsed town phenom thrown back from an earlier breed of vagabond, Crazy Old Man So-and-so. They'll tell stories of the old man who wanders the state land, and on certain summer nights, teenagers will imagine sprightly fiddle tunes echoing across the reservoir for decades after his demise. He will probably miss eating meat, drinking beer, hot water, and talking to people, but of course, I'll know some drop-off points, and will check in regularly (beneath the notice of the Law, not to mention well-meaning relatives), as he fulfills a final lonely dream of mapping the details of the geological and rotted-out cultural landscape of what minor folds of southern New England forest as remain.

Hey, if the old man can fantasize about doing it, I can fantasize about enabling it. I'm rooting for anyone who can buck the trend and reinvent their existence a little, on their own terms. In my dad's case, however, it's hard to imagine a good way to cross the arc from civilization and family to a solitary hut in the woods.

In the old neck of the woods (so to speak) where I grew up, Dad would be following some of the footsteps of at least one famous old eccentric, who camped in a number of wooded caves (less wooded than now). The Leatherman was one of those nameless drifters, special in that he managed to acquire local acclaim, forever making a clockwork circuit of southwestern Connecticut and eastern New York, covering the 365-mile loop in a dependable month, regardless of the weather. His nickname name came from his obviously home made clothing, and his caves have since been discovered, and named. One of them is a pleasant hike not far from my parents'.

The Leatherman must have suited the contemporary conception of an 1860s vagabond, must have touched something that otherwise felt lost to the urbanites and farmers of his day. Or maybe it was just the fact that he showed up so regularly that his returning presence was comforting to the locals. The Leatherman was given special dispensation to roam through the towns who'd otherwise outlawed vagrancy, and he was the frequent recipient of charity, gifts of food received on the back steps, and frequently eaten in the presence of the givers. Sometimes he bought supplies, using unknown means.

A local paper gave the Leatherman a proper name, and storywhich looks for all the world like some crappy Victorian melodrama. If you confine your reading to nineteenth century novels, you'll take home a mixed view of madness. Idiocy wasn't much loved, but there was endless intrigue in what makes a human let go of worldly associations, some bittersweet drama of betrayal or trauma. And the American stories were brimming with rugged individualists and wandering frontiersman. During a forced hospital stay, the Leatherman was pronounced "sane but emotionally afflicted," whatever the hell that means. I have to imagine there was a lot of emotional affliction seething just under the surface in those years, among that surviving generation. Even in the best of times, to be alive is to be emotionally afflicted.

The economic shakeups of the Civil War and industrialization made for lots of vagrants in the Leatherman's time, and a new means of travel got them a new name, but with an exception or two, that shit obviously got old soon enough in gentle society, to the point of public complaint about the tramp problem. (I think it took a big injection of the recently stable into the ranks to bring a measure of romance to the life of the Depression-era hobo.) The Leatherman's days must have had many less-loved wanderers and hermits floating around. The public attachment to this man, must have been tied up in what literary torment he invited. We seem more fascinated with the voluntary outcasts, with those willing to go feral by conviction or by choice. I guess the more mundane tragedies that force a body into rugged individualism don't carry the same dramatic depth as the martyr's self-imposed exile. In the case of the itinerant tramps, there was an issue of strangeness that the Leatherman had overcome. But did the mere wanderers-about-town enjoy the same kind of charity he did? Did the madmen holing up the town woods? Did the urban homeless?

And you know, there's always a temptation to look at the road ahead and just keep going. And always a good reason to turn back. The woods thing is my Dad's unlikely fantasy--I'm still working out my own pointless cubicle dreams, which involve a more urbane sort of withdrawal, a less dramatic restatement of principles. I'll let you know if I figure it out.



(Here's a bonus documentary on the leather dude. Stay away from tobacco, kids.)

Crepuscular

If you're ever lacking evidence that the world is a set, that it's some elaborate and nearly-convincing ruse built to test your endurance against injustice, uncertainty, and some unconscionable proposition of inevitability (and who's testing, you might ask, and isn't the whole experiment more than a little unethical, and what the fuck, why me anyway, who does that make me in this whole ridiculous equation? ...well, never you mind about any of that), then the place to look is up. Sunbeams stretch out misleadingly from breaks in the clouds, and spread like divine fingers to the earth, or maybe like some benevolent or retributive holy gaze. I've often taken sunbeams reaching through the clouds as a meaningful representation of God, casting His beatific essence from the sky to the earth, a view supported by a wasted youth of studying comic illustrations.

But it's a fake, right? A prop? If you think about the alleged distance of the sun to the earth, not to mention the relative diameters of the bodies, then we're talking a mighty slim solid angle. These photons are clearly jetting down to the little blue globe like soldiers in a column, marching at lightspeed to cross this uniquely flyblown orb all in line. That's the story, anyway, and I call bullshit on it. It's bad enough that y'all act so unconvincingly, but at the right time of day, the awkward backlights couldn't be more obvious. What else am I supposed to think? And if it looks bad on the ground, get up in an airplane at one of the crepuscular hours, and witness, motherfucker. The sunbeams splay out from a dozen spotlights that are plugged in at various points only just barely behind the clouds, each converging at its own infinity, and not a common one. I can only believe they're quietly clicked off in sequence by the Cosmic Designers when night is designated to commence. In any case, wherever the beams finally do co-locate, it's some point a lot closer than a hundred-million-mile-distant perpetual H-bomb. As if.

I expected a more exotic explanation when I finally got around to looking this business up, some unlikely scattering event at the edges of the clouds maybe, or perhaps some ramification of being forced to shine through the dynamic array of cloud droplets. But it's nothing so complex as that. The image of sunbeams is a boring matter of perspective, more like watching the road recede infinitely before you and converge to a point than a sophisticated manipulation of the light. The counterintuitive trick is that the opening in the sky is the size of a New England county, and the sky itself is more enormous that it looks, at least a mile or two distant depending on the altitude of the phenomena. The perspective is greater than it appears, and yet, and yet...

You can spot crepuscular rays whenever light is shining down through a hole in the firmament and there are enough scatterers to make it look like a beam, and, as the word suggests, they're more likely to be observed at the right times of day. I hadn't realized it when I first thought to complain about the phenomenon, but there are anticrepuscular beams up there too, streaks of light pitched clear across the opposite horizon, that complete the other half of the surprising arc, and on rare occasions (I read), you can see the beams stretch across the length of the sky. Great, and now I'm going to be looking for them.

My favorite beams are the ones that streak out right before sunrise and just after sunset, that unitary burst, when a single ray streams vertically from just below the edge of the world, a godly claw reaching up to the sky to carve a path for the rising fireball.

Although the optical effect has grown into its definition, "crepuscular" is an unworthy word for this chunk of atmospheric beauty. It sounds, well, like crap. I can understand the motivation to attach a better significance to the way the light arcs across the sky. Jacob, it's said, could climb the ladder to heaven along these beams, and the Greeks believed (or preferred to believe) that water ascended by these illuminated staircases too. Rain had to come from somewhere, after all. I don't know if the Maori picture of roping back the sun is unique to their culture, but it's the favorite story I've found so far. If the old Polynesians had managed a more developed science of lighting before Whitey stumbled in with the muskets, then maybe they would have picked up on the multiple point sources before I did. I like the idea of lassoing flaming giant back. It's a myth with the right attitude to confront this obnoxious and glorious illusion.

[I had this post in mind before Smutty got going on about paddling the midwest in the prime of his life, but here's a bonus sunburst. His series is a great one. This is one of his photos.]

Review: Call of Cthulhu and Other Weird Stories, by H. P. Lovecraft (edited by S. T. Joshi)

Yes, I have been aware of the hints in that accursed book of the incipient horrors of the sleeping Old Ones, and sure, I too have received witness of His recent presidential campaign (infesting the dreams of sensitives is a less effective campaign tool than phone banking and booking TV spots, turns out), but somehow, for all these years on the internet, I've never managed to pick up any of the source material before now. Now that I've read a collection of Lovecraft short stories and novellas, I can finally get in on the jokes, which, of course, should put an end to their humor once and for all.

As a writer, Lovecraft occupies an interesting transitional space between the nineteenth century horror (he reeks of Poe and Shelley, especially in his earlier stories), and pulp. The usual vibe is that of a dime novel doctored up with a satisfying facsimile of Romantic prose stylings, horror conventions, and histrionics. Throw in some genuine bits of underlying cleverness, a continuity that spans pretty much all the stories, goofy but oddly compelling alien names, and some quality local scenery, and I get the appeal. And Lovecraft does deserve credit for the new directions in which he took the horror genre, replacing the gothic supernatural environment with a malevolent, impersonal science fictional universe, advocating rational inquiry, but leaving the horrors of the cosmos still beyond human explanation, but still looking for it the spooky old places and populating it with scary beings. It makes for a really odd mix: stylistically, thematically, like some kind of nerd horror. I couldn't really let go of the image of a prissy boy Howard writing stern letters to the pulps over their editorial standards; I pictured him tearing through unconventional texts like the young Victor Frankenstein (or his young creature), letting their presumed truth lead to his own bizarre interpretations; I pictured a young traveler defensively clutching his valise to his chest on a train as he follows through with his various New England correspondences.

I loved Lovecraft's old New England--I recognize a lot of the places--but it's an unlikely place to expect to find eldritch beings, having been populated (by people Lovecraft would recognize) for only a couple hundred years. The old places the author knew were the backwoods of the Northeast, and the old people were isolated Puritan farmers mucking about on dilapidated homesteads on the distant outskirts of small towns. Lovecraft frequently tried to work in some metaphysics involving ancestral human memory though, and was completely disinclined to entertain any Christian mythology to develop those ideas of ancient evil (although I suppose The Festival and Nyaralthotep come a little closer than some of them). How well any of these themes work within the limitations of the setting depends on the story, and I think the fact that even early America isn't so very old is what pushed Lovecraft to populate stories with his memorable aliens.

In addition to the contention between setting and theme, Lovecraft also often suffers from deciding badly what to tell and what to show. It's as though he absorbed the lesson that horror is sometimes better hinted at, but it took a lot of practice to get the hang of applying it. I rolled my eyes at all of the unseen and unnameable. When he does decide to describe more, the stories are invariably better. The Colour out of Space* creates the best presence out of the collection, doesn't shy so much from describing the extraterrestrial decay infecting the countryside, logs in the most details of the adversary, and for that matter, captures the Massachusetts countryside best (it's the Quabbin reservoir!), and is easily the scariest and most intriguing entry of the bunch.

The earlier stories don't find their balance as well, and probably the author thought so too, compelled as he was to revamp some of his first takes. The Call of Cthulhu is obviously Dagon rewritten, The Shadow over Innsmouth is Arthur Jermayne with fish instead of apes. Cool Air uses the same ideas in Herbert West: Reanimator to tell a story that doesn't suck so much the second time around. As far as the compilation goes, I don't think it was a good idea to present Lovecraft's work chronologically to new readers. There are a couple decent Poe pastiches early on (The Outsider and The Hound), and the very short pieces present nothing particularly objectionable, but when the editor tells me in interminable footnotes that Lovecraft found this or that story overwritten, I kept thinking, "how could anyone possibly tell?" Lovecraft sure likes to pile on the macabre adjectives, and to a character, every one of his good skeptics is on the verge of suicide in superlative despair. His scientific minds, again like Frankenstein's, were cursed with a curiousity that was unable to bear the revealed evil when it goes too far. (Didn't any of these scientists find some of the less-malevolent aliens just plain intesting? Fuck it, but I would have thought long and hard about putting my brain in a can for a chance to see Pluto.)

When Lovecraft does find a place for all of his diverse influences, he can get a good story out of it, and he does have a fair knack for unsettling dreamlike imagery and for digging deeply into local scenery. It's worth reading, but I'd probably recommend going after the four or five stories in the canon and leaving it at that.






* Although let me say that this business of "no known color" annoyed the hell out of me. Color is that set of electromagnetic radiation that people see, the stuff that is known by definition. Something colorful in some other part of the spectrum doesn't mean it's not colorful in the visible, and people ain't going to see it anywhere else. I'll cut Ambrose Bierce some more slack, but The Damned Thing, which Lovecraft is borrowing from here, and which I've improbably read, was also annoying.